Chaos Painters

By Naomi Mitchell

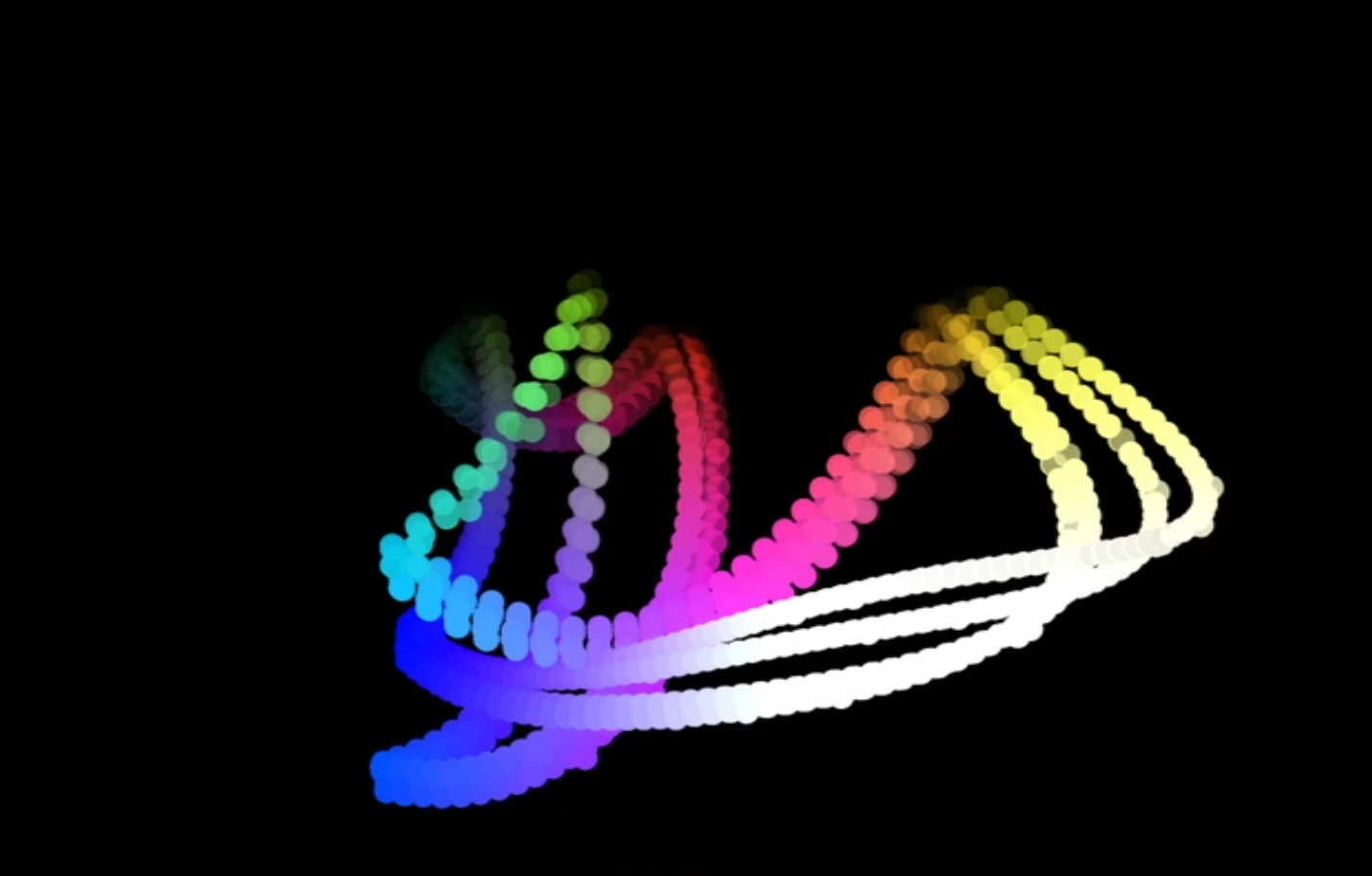
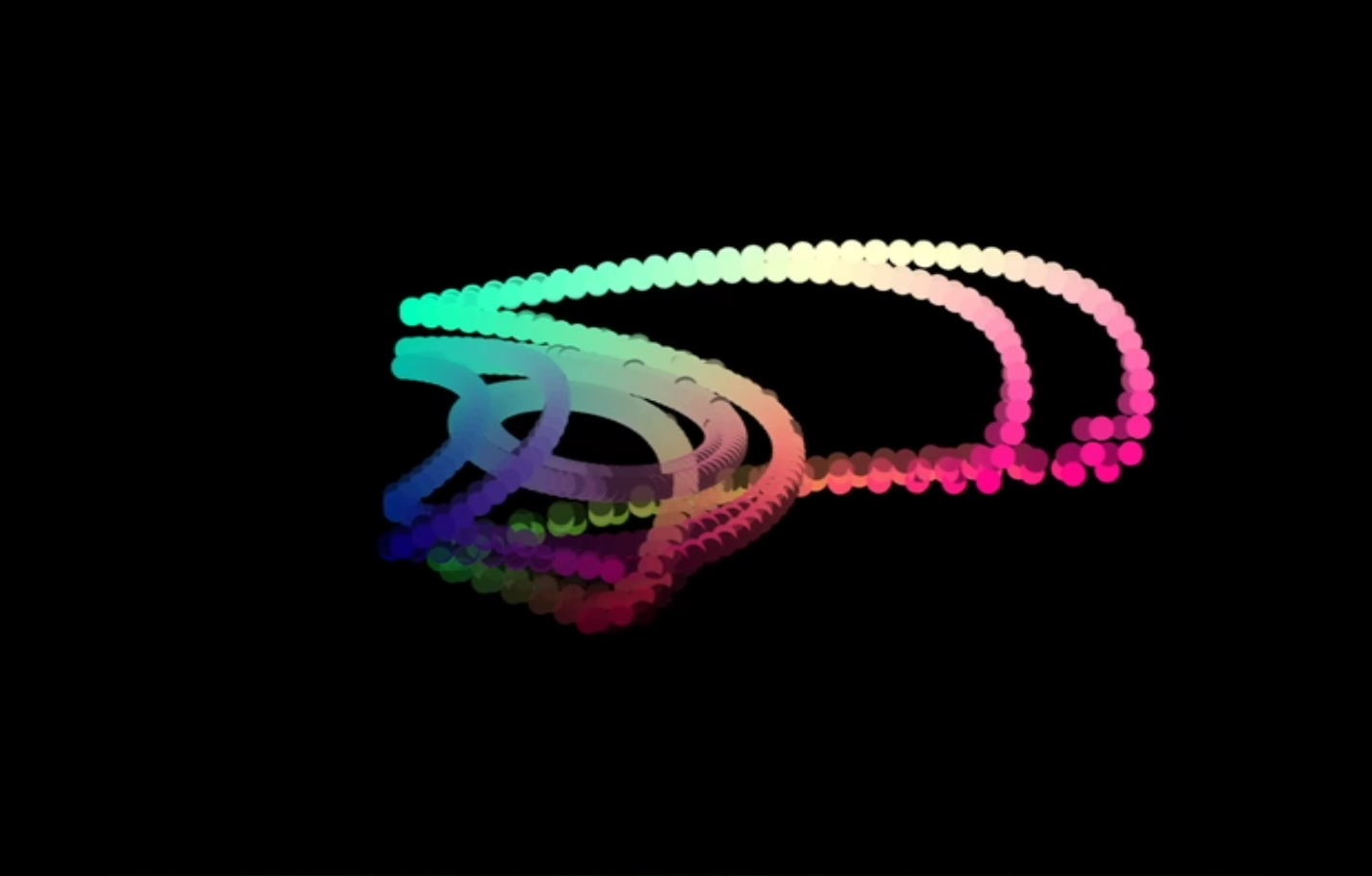
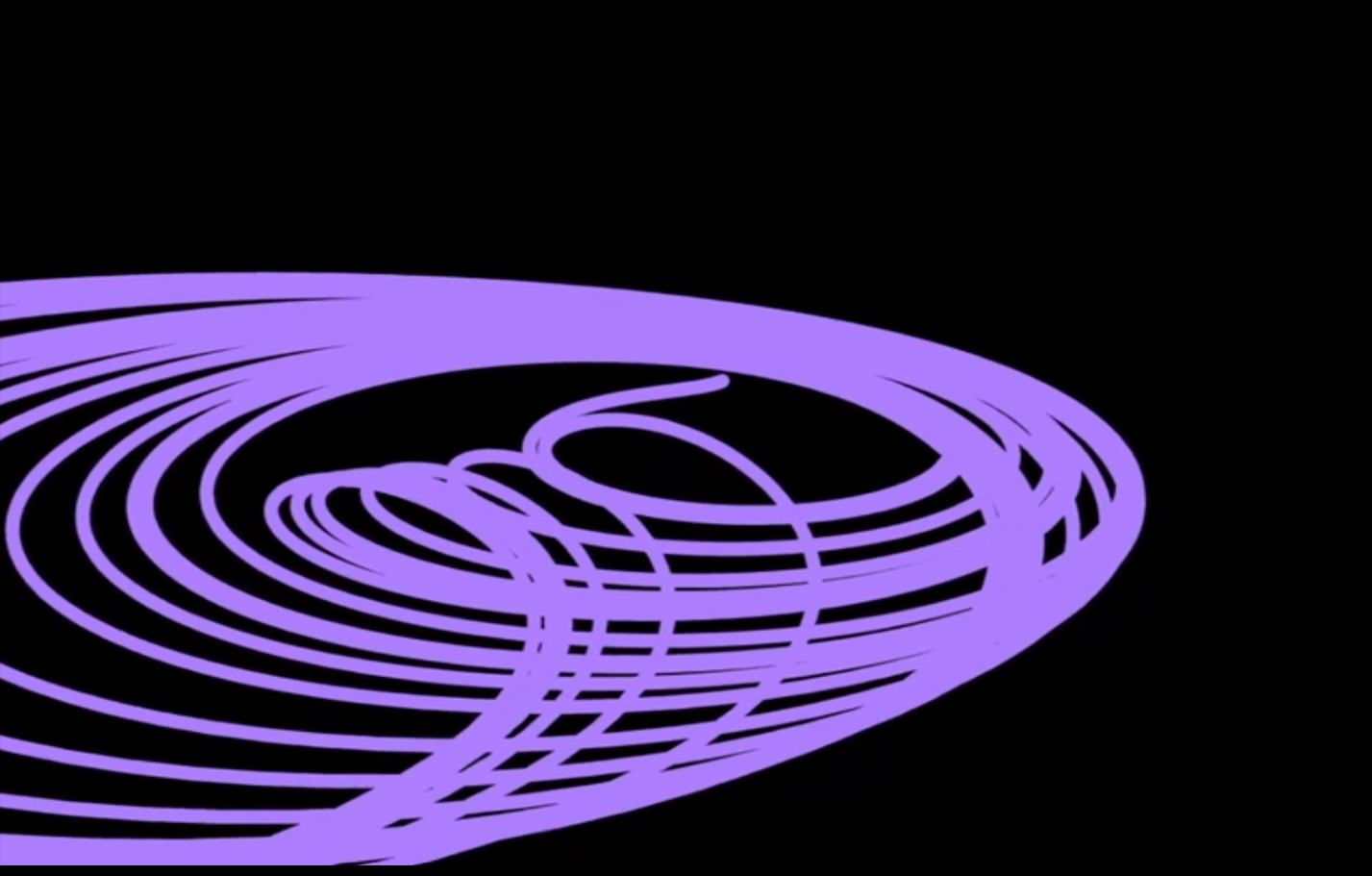
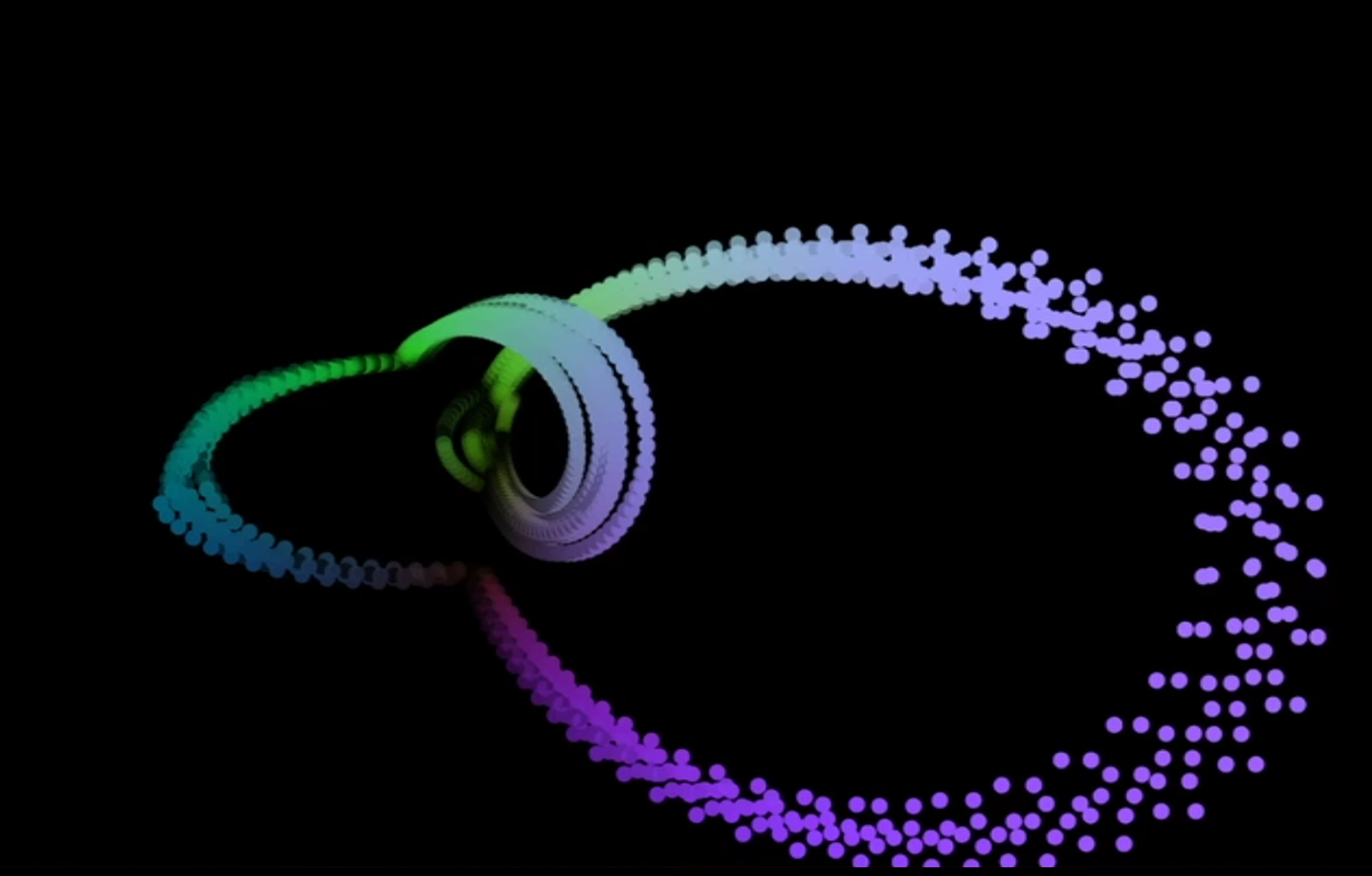
Chaos Painters is an exploration of mapping chaotic differential equations in 3D environments. The 38 clips are split into two different representations of 13 chaotic equations.

$5

From the Artist

The selected chaotic equations are from the work of J.C. Sprott, specifically the paper Some Simple Chaotic Flows. The 19 equations are some of the simplest implementations of chaos, with five composed of five terms and two nonlinearities, and the rest composed of six terms and one nonlinearity. The idea of exploring chaos at the simplest possible level is extremely interesting to me as a researcher and artist. The complexity of chaotic nonperiodic behavior expressed by some of the simplest terms is fascinating. 

The visuals are generated in two ways. Most are generated by a combination of Max/MSP and Processing, where the X, Y, and Z terms of the equations determine both the position and color of the painters. The five others, labeled TD_, were made in a Python script in TouchDesigner and are monochromatic; the X, Y, and Z terms only correspond to the position of the painter, but include rotational camera movement around the painters. This video pack uses 13 of the 19 equations, selected because of their visual interest and successful implementation. 

I examined these equations and other chaotic and pseudo-random phenomenon as part of my master’s thesis, which can be read here